
The Rayleigh ratio (Rq) is defined as the relative scattering intensity or the ratio of scattered (Is) to incident (Io) light, with the strict definition requiring that the scattering intensity be summed over all space. Because of geometric limitations imposed by the practical restriction of measuring the intensity at a given angle (q) and distance (r) from the scattering volume, the working expression for the Rayleigh ratio is that shown below, where is is the scattering intensity measured at fixed r and q.
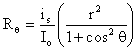
The sample scattering intensity is dependent upon the permittivity (eo) of the medium and the polarizability (a) of the particle being measured, which allows us to express the above equation in the form shown below, were r is the particle density, NA is Avogadroís number, M is the particle molecular weight, and lo is the vacuum wavelength of the incident light.
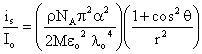
The Rayleigh ratio, can then be defined in the following form.
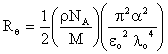
When light interacts with matter, the oscillating electromagnetic wave of the incident light induces an oscillating dipole in the electronic cloud of the molecule. It is the oscillation of the dipole that leads to the light scattering phenomenon. As such, the polarizability of the molecule is representative of the ability of the molecule to scatter light. While it is not a simple task to measure polarizability, the Clausius-Mosetti equation can be used to relate the polarizability to the permittivity of the molecule. After substitution and rearrangement, the Rayleigh ratio equation can be expressed in the form shown below, where C is the weight concentration, M is the weight average molecular weight, A2 is the 2nd virial coefficient (indicative of particle -solvent interactions), K is a constant that embodies all of the optical properties of the solution being measured, Ůo is the solvent refractive index, and dŮ/dC is the specific refractive index increment for the particle Ė solvent pair.
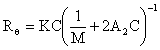
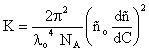
As evident in the above expressions, the Rayleigh ratio is dependent upon the particle concentration and molecular weight. It is this dependence that is utilized for static light scattering molecular weight measurements of small particles. Rearrangement of the above expressions gives the more commonly known form of the Rayleigh equation shown below.
![]()
While the definition of the Rayleigh ratio (is/Io) implies a straight forward means of measurement, in practice, it is difficult to measure Io and r with the precision necessary for accurate determination of the sample Rayleigh ratio. A more commonly used approach is to compare the scattering intensity of the sample to that of a well characterized reference, such as toluene, with a known Rayleigh ratio. The sample scattering intensity can be described by rearrangement of Equation 1.
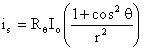
If the scattering intensities of the sample and the toluene reference are measured under the same conditions, the sample Rayleigh ratio can be determined from the ratio of scattering intensities as shown below, where the A and T subscripts represent the analyte and toluene respectively and the Ů terms are included to account for differences in the refractive indices of the toluene and sample solutions.

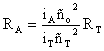
Since it is the photon count rate (CR) rather than the scattering intensity that is measured at the detector in typical light scattering instrumentation, the expression for RA is converted to the working equation shown below.

For additional questions or information regarding Malvern Instruments complete line of particle and materials characterization products, visit us at www.malvern.com.
